Cottrell equation
Electrochemical reactions can be limited in multiple ways. The two main limitations are potential and diffusion.
The potential is the driving force of a reaction, which is described by the Nernst Equation. If the potential is high enough to drive a reaction, another problem occurs: Depletion.
The reaction occurring at the electrode consumes an active species in front of the electrode. This active species is then depleted and is missing for the reaction to occur. New active species is transported to the electrode by diffusion. Now the diffusion is determining the current flowing at the electrode.
The change of the current due to depletion and diffusion after a potential step is described by the Cottrell equation:
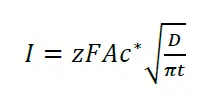
Here I is the current, z the number of transferred electrons, F the Faraday constant, A the surface area of the electrode, c* is the bulk concentration, D the diffusion coefficient and t the time.
Articles

The Cottrell Experiment and Diffusion Limitation – PDF
This PDF provides the instructions for the experiment related to the article series 'The Cottrell Experiment and Diffusion Limitation'. Have fun!

The Cottrell Experiment and Diffusion Limitation 3/3 – Electrochemical Double Layer
In this chapter the electrochemical double layer and its features are discussed. The electrochemical double layer acts as a capacitor and every change in the potential of the electrode will induce a capacitive charging current that is caused by physics not by a chemical reaction. This current decays exponentially.
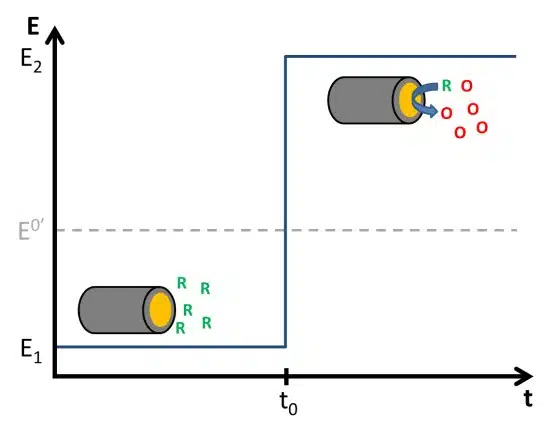
The Cottrell Experiment and Diffusion Limitation 2/3 – The Cottrell Experiment
In this chapter, we discuss the Cottrell experiment. During the Cottrell experiment a potential step across a redox potential is applied and the current caused by the electrochemical reaction is recorded. The current is controlled by diffusion.

The Cottrell Experiment and Diffusion Limitation 1/3 – Introduction
This series of articles aims to provide an introduction to electrochemical experiments. This series in particular deals with the Cottrell experiment and diffusion limitation, and includes an experiment (PDF) you can carry out yourself.
