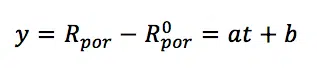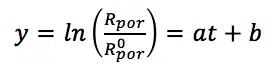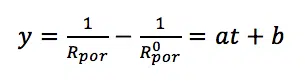Coating Research in Food Industry
In this section it is explained why Electrochemical Impedance Spectroscopy (EIS) is a suitable technique to perform research on coatings meant for food storage. Furthermore, it will be explained how to estimate the time until an unacceptable resistance of the coating is reached based on EIS measurements. During the calculations also the order of the reaction leading to the failure of the coating and the reaction rate will be determined.
Predicting the expiration date of food containers
Coating for food storage containers or other objects that get in contact with food is important to prevent spoiling or contamination of the food as well as to protect the container itself from corrosive properties of the food. Tins are still quite common as food containers, due to the fact that the food is protected from air and light for a long time. Tins are conducting surfaces, which allow electrochemical studies of themselves and their coatings.
In an ideal case the expiration date stored in a food container can be predicted. Possible obstacles for a prediction are the different reaction rates of the spoiling components of the food, which are not always constant. Often the reaction rate is linear or a parable. Furthermore, the storage temperature might be different, for example if the food is exported to different countries.
Measurements at different temperatures and storage times
As discussed in previous chapters a high polarization resistance or the resistance added by the coating means a low corrosion of the can, which means a longer shelf life for the food contained in it. The resistance of the coating is as well influenced by the temperature and the storing time. To make a proper prediction of the shelf life, measurements performed at different temperatures and storage times will be needed.
EIS, as a non-destructive technique with high sensitivity for interface changes, is a suitable method to investigate coatings for food cans. It is assumed that you are familiar with the principles explained in previous chapters, so this won’t be discussed here.
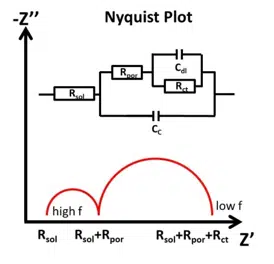
Most coatings will behave like an ideal coating directly after filling the can, which means that they are a resistor and capacitor in series (see Figure 6.5). More complex behavior will be developed over time due to water penetrating the coating. As long as no corrosion has started, the EIS will look like a simplified Randles circuit (Figure 6.7). The serial resistor would stay the Rsol, but the charge transfer resistor Rct would be replaced by the pore resistance Rpor and the double layer capacity Cdl by the coating capacitance CC.
When the water reaches the metal interface, corrosion can appear. This situation is reflected by the equivalent circuit in Figure 8.1. The corrosion can lead to gas formation under the coating, leading to blisters and disbonding. The solution inside a blister, as long as the blister is closed, can be quite different from the bulk solution. The corrosion reactions often lead to a low pH value.
Testing the Shelf Life
First, cans are coated and packed as usual. The cans are tested after certain time periods, for example 1 day, 1 week, 1 month, 4 months and 12 months.
If the product in the can is conducting, the measurement can be performed in the product, which acts as an electrolyte. Otherwise, the product needs to be replaced with 0.5 M NaCl solution. Keep in mind that a fat or oil film on the can coating might act as an additional film, but there is also the possibility that strong detergents to remove the fat film will change your coating.
The can is connected as the working electrode. The reference and counter electrodes are immersed in the can’s solution. Electrochemical Impedance Spectroscopy is performed and the spectra for the different storage times compared. It is expected that Rpor will decrease over time, when the quality of the film decreases.
As stated previously there is a correlation between the quality of a coating and how fast food spoils. It seems there is a connection between the pore or coating resistance and the reaction rate r of the spoiling substances. The reaction rate r is defined as the negative change of the reactant’s concentration. The change depends on the concentration of the reactant to the power of n and the reaction rate constant k. Common values for n are 0, 1 or 2 (see equation 8.1).
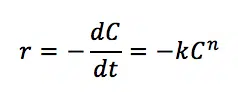
Using the Power law we can exchange the concentration C for the pore resistance Rpor (Equation 8.2).
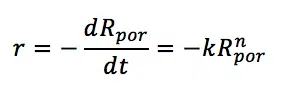
If n is defined and the behavior of k is understood, the reaction rate after long time periods can be extrapolated. Determining n and k is best done by transforming the differential equation for each of the three common n (0, 1, and 2) into a linear relationship. Sample data is plotted according to these linear relations and via a linear fit it is determined which order fulfills best the linear criteria.
Solving the zero-order equation delivers
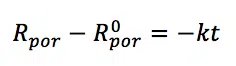
R0por is the pore resistance at day 0. If the difference between Rpor and R0por is plotted versus the time t and a linear relationship is visible, the reaction is 0 order (n = 0) and the slope of this curve is –k.
The solution of the 1st order (n = 1) delivers
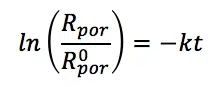
A plot of the ratio’s logarithm versus the time t should deliver a linear curve with a slope of –k, if the reaction is of 1st order.
Analog the 2nd order (n = 2) equations deliver
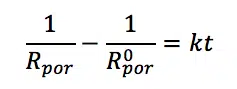
In case that the reaction is of 2nd order a plot of inverses’ difference versus the time t will deliver a linear curve, but this time the slope is k (not –k). The fit with the best correlation coefficient R² is made with the correct n.
This way the reaction order as well as the reaction rate can be determined. With the values from the linear fit the time t it takes to reach a certain Rpor can be calculated. The linear fit result is described by equation 8.6.
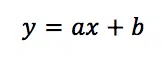
With the slope a and the intercept b. If you keep in mind that x for this case is t, the equations needed to calculate t for the different n are:
If the time t, where the can isn’t acceptable anymore needs to be determines, an Rpor which isn’t acceptable anymore needs to be defined, for example 300 Ω. With the R0por from the measurements and the afterwards determined n and k, the time t until the non-acceptable Rpor is reached can be calculated. After a and b have been determined from the linear fit, the corresponding equation (8.7, 8.8, or 8.9) is rearranged for t.
With this method the behavior over time for a fixed temperature can be predicted, if several values at different points in time are known. If the reaction rates for multiple temperatures are known, the reaction rates and shelf lives for other temperatures can be extrapolated. However, the extrapolations are to be taken with a pinch of salt. Changing the temperature might change other factors that influence the reaction rate k. Phase transitions can cause these changed, for example.
In food packaging the glass transition is an important influence. Due to the complexity of this topic, we recommend that readers, who want to determine from reaction rate or pore resistance measurement the shelf life for a temperature that wasn’t used in the measurements, to consult the corresponding literature.